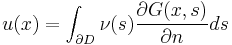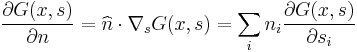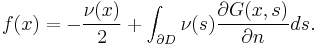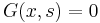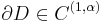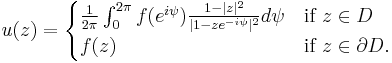Dirichlet problem

In mathematics, a Dirichlet problem is the problem of finding a function which solves a specified partial differential equation (PDE) in the interior of a given region that takes prescribed values on the boundary of the region.

The Dirichlet problem can be solved for many PDEs, although originally it was posed for Laplace's equation. In that case the problem can be stated as follows:

Given a function f that has values everywhere on the boundary of a region in Rⁿ, is there a unique continuous function u twice continuously differentiable in the interior and continuous on the boundary, such that u is harmonic in the interior and u = f on the boundary?

This requirement is called the Dirichlet boundary condition. The main issue is to prove the existence of a solution; uniqueness can be proved using the maximum principle.

1 History
2 General solution
- 2.1 Existence
3 Example: the unit disk in two dimensions
4 Generalizations
5 See also
6 References
7 External links

History

The Dirichlet problem is named after Lejeune Dirichlet, who proposed a solution by a variational method which became known as Dirichlet's principle. The existence of a unique solution is very plausible by the 'physical argument': any charge distribution on the boundary should, by the laws of electrostatics, determine an electrical potential as solution.

However, Weierstrass found a flaw in Dirichlet's argument, and a rigorous proof of existence was found only in 1900 by Hilbert. It turns out that the existence of a solution depends delicately on the smoothness of the boundary and the prescribed data.

General solution

For a domain 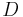$D$ having a sufficiently smooth boundary 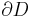$\partial D$ , the general solution to the Dirichlet problem is given by

$u(x)=\int_{\partial D} \nu(s) \frac{\partial G(x,s)}{\partial n} ds$

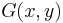where $G(x,y)$ is the Green's function for the partial differential equation, and

$\frac{\partial G(x,s)}{\partial n} = \widehat{n} \cdot \nabla_s G (x,s) = \sum_i n_i \frac{\partial G(x,s)}{\partial s_i}$

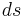is the derivative of the Green's function along the inward-pointing unit normal vector $\widehat{n}$ . The integration is performed on the boundary, with measure $ds$ . The function $\nu(s)$ is given by the unique solution to the Fredholm integral equation of the second kind,

$f(x) = -\frac{\nu(x)}{2} %2B \int_{\partial D} \nu(s) \frac{\partial G(x,s)}{\partial n} ds.$

The Green's function to be used in the above integral is one which vanishes on the boundary:

$G(x,s)=0$

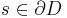for $s\i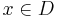n \partial D$ and $x\in D$ . Such a Green's function is usually a sum of the free-field Green's function and a harmonic solution to the differential equation.

Existence

The Dirichlet problem for harmonic functions always has a solution, and that solution is unique, when the boundary is sufficiently smooth and 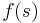$f(s)$ is continuous. More precisely, it has a solution when

$\partial D \in C^{(1,\alpha)}$

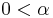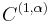for $0<\alpha$ , where $C^{(1,\alpha)}$ denotes the Hölder condition.

Example: the unit disk in two dimensions

In some simple cases the Dirichlet problem can be solved explicitly. For example, the solution to the Dirichlet problem for the unit disk in R² is given by the Poisson integral formula.

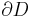If $f$ is a continuous function on the boundary $\partial D$ of the open unit disk 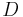$D$ , then the solution to the Dirichlet problem is 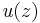$u(z)$ given by

$u(z) = \begin{cases} \frac{1}{2\pi}\int_0^{2\pi} f(e^{i\psi}) \frac {1-\vert z \vert ^2}{\vert 1-ze^{-i\psi}\vert ^2} d \psi & \mbox{if }z \in D \\ f(z) & \mbox{if }z \in \partial D. \end{cases}$

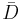The solution $u$ is continuous on the closed unit disk $\bar{D}$ and harmonic on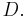 $D.$

The integrand is known as the Poisson kernel; this solution follows from the Green's function in two dimensions:

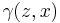$G(z,x) = -\frac{1}{2\pi} \log \vert z-x\vert %2B \gamma(z,x)$

where $\gamma(z,x)$ is harmonic

$\Delta_x \gamma(z,x)=0$

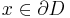and chosen such that $G(z,x)=0$ for $x\in \partial D$ .

Generalizations

Dirichlet problems are typical of elliptic partial differential equations, and potential theory, and the Laplace equation in particular. Other examples include the biharmonic equation and related equations in elasticity theory.

They are one of several types of classes of PDE problems defined by the information given at the boundary, including Neumann problems and Cauchy problems.

References

A. Yanushauskas (2001), "Dirichlet problem", in Hazewinkel, Michiel, Encyclopedia of Mathematics, Springer, ISBN 978-1556080104, http://www.encyclopediaofmath.org/index.php?title=d/d032910
S. G. Krantz, The Dirichlet Problem. §7.3.3 in Handbook of Complex Variables. Boston, MA: Birkhäuser, p. 93, 1999. ISBN 0-8176-4011-8.
S. Axler, P. Gorkin, K. Voss, The Dirichlet problem on quadratic surfaces Mathematics of Computation 73 (2004), 637-651.
Gilbarg, David; Trudinger, Neil S. (2001), Elliptic partial differential equations of second order (2nd ed.), Berlin, New York: Springer-Verlag, ISBN 978-3-540-41160-4